Poisson Regression
YouTube search... ...Google search
- AI Solver ... Algorithms ... Administration ... Model Search ... Discriminative vs. Generative ... Train, Validate, and Test
- Regression Analysis
- Math for Intelligence ... Finding Paul Revere ... Social Network Analysis (SNA) ... Dot Product ... Kernel Trick
- Poisson Regression? | Microsoft
- Choosing the Correct Type of Regression Analysis | Jim Frost
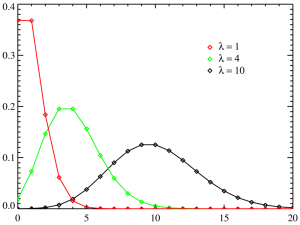
Poisson regression is used to model response variables (Y-values) that are counts. It tells you which explanatory variables have a statistically significant effect on the response variable. In other words, it tells you which X-values work on the Y-value. It’s best used for rare events, as these tend to follow a Poisson distribution (as opposed to more common events which tend to be normally distributed). For example:
- Number of colds contracted on airplanes.
- Number of bacteria found in a petri dish.
- Counts of catastrophic computer failures at a large tech firm in a calendar year.
- Number of 911 calls that end in the death of a suspect.
For large means, the normal distribution is a good approximation for the Poisson distribution. Therefore, Poisson regression is more suited to cases where the response variable is a small integer.
Poisson regression is only used for numerical, continuous data. The same technique can be used for modeling categorical explanatory variables or counts in the cells of a contingency table. When used in this way, the models are called loglinear models. What is Poisson Regression? | Statistics How To